Pattern formation of active surfaces immersed in viscous fluids
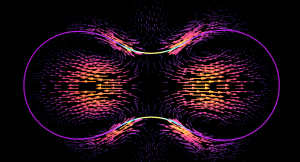
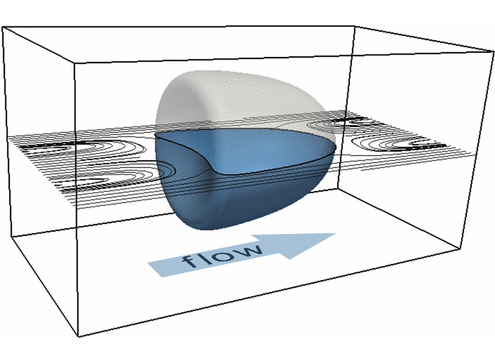
The formation of a contractile rings along an active surface driven by the positive feedback loop of surface flows and surface protein concentration. Rings are unstable and slip away.
The surface of biological cells is connected to the cell cortex – a thin layer of active material. This layer exerts an active contractile tension which regulates cellular shape by deforming the surface. The strength of the active tension is controlled by the concentration of force-generating molecules. Advective transport of these molecules leads to a complex interplay of surface deformation, hydrodynamics and molecule concentration which gives rise to pattern formation and self-organized shape dynamics. We develop an arbitrary Lagrangian-Eulerian model to simulate such an active viscous surface immersed in viscous fluids. In collaboration with bio-physicists we analyze the patterning, cell shape dynamics and flow profiles to better understand the functioning of biological cells.
Funded by: DFG (AL1705/6)
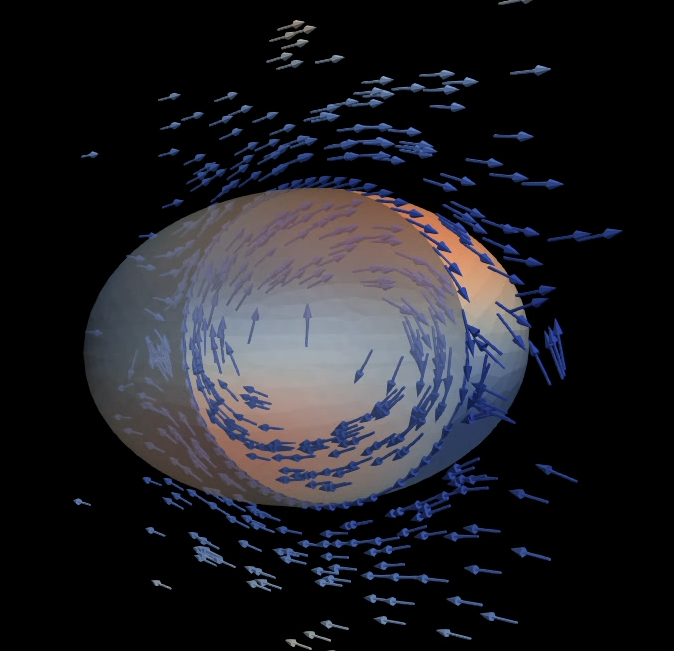
Numerical Simulation of novel Microswimmers
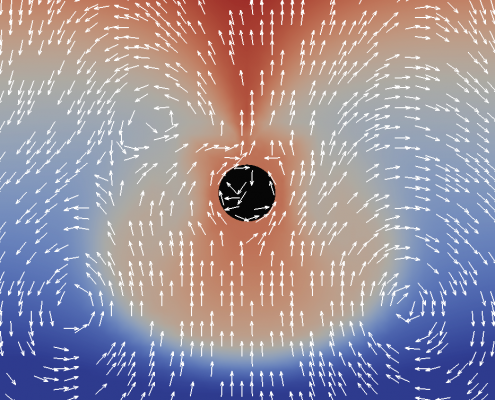
An elastic shell swims though a viscous fluid by periodic a buckling instability triggered by pressure oscillations
The creation of microscopic robots that can swim through a liquid environment is a very active branch of research. Given the increasing miniaturization, such microswimmers could fulfill many useful task, the most prominent of which being targeted drug delivery by swimming through blood vessels, even against the flow.
Using numerical simulations, we help developing a new mechanism for microscopic, swimming robots. The mechanism is based on a buckling instability of air-filled elastic balls. These balls can be excited to periodic swimming motion by oscillatory changes of the surrounding fluid pressure (achieved by ultrasound waves). Together with researchers of the french Université Grenoble Alpes, we use simulations to improve understanding of this new swimming technique. Simulations are used to virtually predict and optimize the swimming properties and to explore potential applications.
Wetting of structured and elastic surfaces
Simulation of a droplet rolling of a surface covered with elastic micropillars
The Lotus effect is one of the most prominent examples where nanotechnology has learned from nature. Nowadays, industrial products are equipped with microscopic structures on their surface that mimic the Lotus flower’s ability to repel water and clean itself. But nanotechnology aims to go further to create robust oil-repelling structures, which would lead to surfaces that clean themselves even from oily films (imagine self-cleaning glasses and windows). To this end, special surface structures on the length scale of micrometers are necessary, but as these are very thin, they will elastically deform as soon as they get in contact with a fluid interface. The interplay of thin structures with fluids on small length scales is to date almost unexplored.
We develop improved phase-field models to simulate the wetting of elastic nano-structured surfaces. Particular focus is on the stable coupling of the tight interplay of capillary forces and structure elasticity. Together with experimental partners we explore what happens when liquid droplets slide over elastic and microstructured surfaces and reveal how nearby droplets interact with each other by deforming the surface. We aim to get a fundamental understanding of these processes setting basis for future emerging technologies.
Funded by: DFG (AL1705-5)
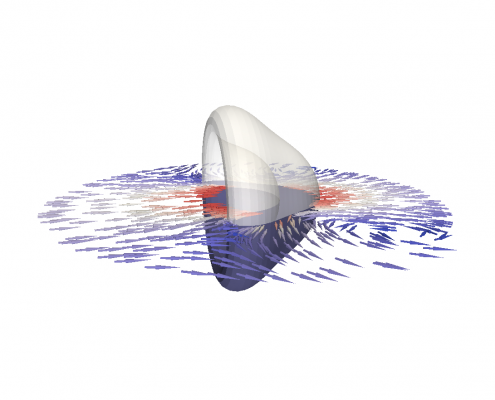
Modeling and simulation of viscoelastic surfaces
An viscoelastic surface (e.g. cell cortex) immersed in viscous fluids undergoes a deformation by shear flow.
The membrane of biological cells is supported by a thin underlying network of microscopic filaments. These filaments are intertwined and attached to each other by proteins, making the membrane resilient against deformation. This network is called the cortex of the cell. It gives the cell membrane an elastic property, making it return to its original shape after being deformed. However, for long lasting deformations, the membrane shows fluidic properties. For prolonged deformation, the proteins connecting the filaments change position. This changes the cortex and with it, the shape of the cell membrane. To simulate the mechanics of both properties we construct the first numerical model of a visco-elastic surface.
The model can be used for realistic simulations of cell mechanics and pave the way for a better understanding of cell shape regulation, cortical pattern formation and surface flows during cell division.
Funded by: DFG (AL1705-6)
Biological cells in flow
Biological cell flowing through a microscopic channel. The cell is modeled by a phase-field model as a neo-Hookean elastic material with surface tension.
The stiffness of biological cells can be used to infer useful information on the cell’s phenotype, state and medical condition. Accordingly, measuring cell stiffness is an important part of biological research with diverse applications in biology, biotechnology and medicine. Reliable and fast measurements are only possible by a connection of high-throughput experimental techniques in combination with numerical simulations. In a new and extremely efficient technique (called RT-DC) cells are flushed through a microfluidic channel and camera images of cell deformation provide an indicator for cell stiffness.
To extract the cell mechanical parameters, cell deformation images must be matched with numerical simulations of the process. To this end, we develop new mathematical models to simulate biological cells in flow. Thereby the cell is modeled as a viscoelastic material surrounded by a thin elastic shell cortex, subject to bending stiffness and cortical surface tension. In collaboration with experimentalists, the project results are used for ultra-fast cell diagnosics to detect diseases and to evaluate new medical treatments.
Funded by: DFG (AL1705-3), Saxonian ministry of Science and Arts
Marangoni flows at fluidic interfaces
We simulate mass transport of surfactants along and across fluidic interfaces. In this process, the so-called Marangoni effect induces a tangential surface flow, which backcouples to the surfactant mass transport. This interplay of transport an flow leads to interesting phenomena and can be used, for example, for new chemical computing circuits.
Funded by: DFG (AL1705-1)
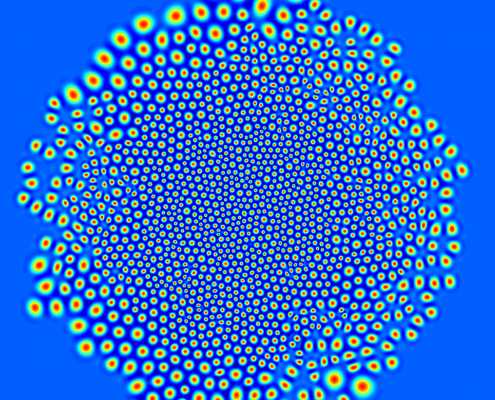
Growth of cell colonies
We investigate the growth of cell colonies by cell division. To this end, we developed a biological Phase-Field-Crystal model that describes cells as freely moving, elastic particles that can grow and divide dependent on mechanical pressure.
Numerical simulations are used to understand the interplay of cell mechanics and growth.
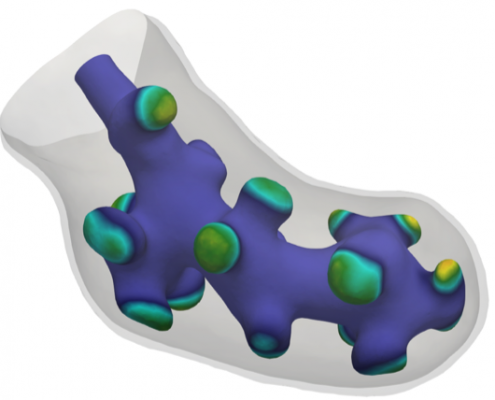
Simulation of lung development
During genesis of animal lungs, a simple tubular embryonic lung evolves to a complicated, multiply-branched shape.
Together with Dagmar Iber (ETH Zürich) we have developed a new mathematical model to describe this evolution The model is based on the interaction of proteins on the embryonic lung surface, leading to a growth complex that enhances local growth.